A quantum computer works by air to solve the factoring

He. She

Edited by Tech Innovation Website – 05/05/2023

Data from each process is stored as base states of qubits, allowing them to be reversed.
[Imagem: Cortesia MaLab/Nanjing University]

Reverse accounts

Austrian researchers have created a new type of quantum computer that runs on air, and reverses the calculations, leaving the results to access transactions.

This is revolutionary for one of the most computationally intensive and widely used tasks today: factorization, used in optimization problems, cryptography, prime number arithmetic, and countless other uses.

Traditional electronic computers are based on processors formed by logic gates. The gate can be, for example, an AND operation, that is, an operation that adds two bits. These gates are irreversible, that is, algorithms cannot simply work backwards.

Professor Wolfgang Lechner of the University of Innsbruck explains.

However, if it were possible, it would be possible to factor large numbers, that is, to factor them, which would greatly reduce the computational overhead, since it would not be necessary to test each of the alternatives until the correct coefficients were found.

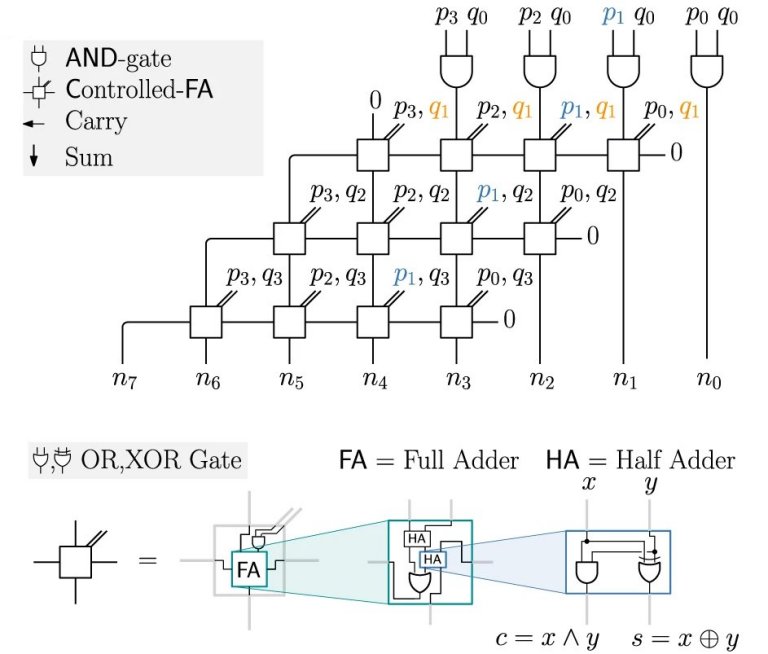
Transactions and results of a traditional logic gate are transferred to qubits.
[Imagem: Martin Lanthaler et al. – 10.1038/s42005-023-01191-3]

quantum factor

The Austrian team has now developed a technique that makes it possible to perform this reversal of algorithms using a quantum processor.

The starting point is the classic logic circuit, the traditional logic gate that multiplies two digits. If two integers are entered as the input value, the circuit returns its output – this circuit is still capable of only irreversible operations.

“However, the circuit logic can be encoded in the ground states of a quantum system,” explained team member Martin Lanthaler. Thus, both multiplication and factoring can be understood as ground-state problems and solved using quantitative optimization methods.

For this, the multiplication data is transferred to qubits of the quantum processor, which stores it as the ground state of each qubit. In this way, to reverse the operation, it is sufficient to find the ground state of each qubit again, which can be performed even after the operation has been performed.

“The core of our work is to encode the building blocks of a multiplier circuit, specifically gates, half gates, and full adders, with the valence structure as a ground state problem, [o que feito] in a set of interactive courses,” Lanthaler explains.

The team’s proposed implementation scheme would work on any quantum computer.
[Imagem: Martin Lanthaler et al. – 10.1038/s42005-023-01191-3]

It works on any quantum computer

The encoder allows the entire circuit to be built from simple subsystems, which are basic building blocks that can be reproduced and arranged in a two-dimensional matrix—to solve larger problems, it is sufficient to assemble more of these subsystems.

Thus, instead of the classical brute-force method, as in current computers, with all possible factors that need to be tested sequentially, the quantum method greatly speeds up the search: to find the ground state of qubits and thus solve an optimization problem, for example , It is not necessary to search the entire energy landscape of qubits, but only to search for valleys, which can be done by the quantum tunneling phenomenon.

According to the team, this equivalence architecture can be implemented on all quantum computing platforms available today.

Other news about: